As the rivalry between quantum and classical computing intensifies, scientists are making unexpected discoveries about quantum systems.
Classical computers outperformed a quantum computer in simulations of a two-dimensional quantum magnet system, showing unexpected confinement phenomena. This discovery by Flatiron Institute researchers redefines the practical limits of quantum computing and enhances understanding of quantum-classical computational boundaries.
Classical Computer Triumphs Over Quantum Advantage
Earlier this year, researchers at the Flatiron Institute’s Center for Computational Quantum Physics (CCQ) announced that they had successfully used a classical computer and sophisticated mathematical models to thoroughly outperform a quantum computer at a task that some thought only quantum computers could solve.
Now, those researchers have determined why they were able to trounce the quantum computer at its own game. Their answer, presented on October 29 in Physical Review Letters, reveals that the quantum problem they tackled — involving a particular two-dimensional quantum system of flipping magnets — displays a behavior known as confinement. This behavior had previously been seen in quantum condensed matter physics only in one-dimensional systems.
This unexpected finding is helping scientists better understand the line dividing the abilities of quantum and classical computers and provides a framework for testing new quantum simulations, says lead author Joseph Tindall, a research fellow at the CCQ.
Clarifying Quantum Boundaries
“There is some boundary that separates what can be done with quantum computing and what can be done with classical computers,” he says. “At the moment, that boundary is incredibly blurry. I think our work helps clarify that boundary a bit more.”
By harnessing principles from quantum mechanics, quantum computers promise huge advantages in processing power and speed over classical computers. While classical computations are limited by the binary operations of ones and zeros, quantum computers can use qubits, which can represent both 0 and 1 simultaneously, to process information in a fundamentally different way.
Quantum technology is still in its infancy, though, and has yet to convincingly demonstrate its superiority over classical computers. As scientists work to figure out where quantum computers might have an edge, they’re coming up with complex problems that test the limits of classical and quantum computers.
Challenging Quantum Supremacy
The results of one recent test of quantum computers came out in June 2023, when IBM researchers published a paper in the journal Nature. Their paper detailed an experiment simulating a system with an array of tiny flipping magnets evolving over time. The researchers claimed that this simulation was only feasible with a quantum computer, not a classical one. After learning about the new paper through press coverage, Tindall decided to take up the challenge.
Tindall has been working with colleagues over the last several years to develop better algorithms and codes for solving complex quantum problems with classical computers. He applied these methods to IBM’s simulation, and in just two weeks he proved he could solve the problem with very little computing power — it could even be done on a smartphone.
“We didn’t really introduce any cutting-edge techniques,” Tindall says. “We brought a lot of ideas together in a concise and elegant way that made the problem solvable. It was a method that IBM had overlooked and was not easily implemented without well-written software and codes.”
Exploring Quantum Confinement
Tindall and his colleagues published their findings in the journal PRX Quantum in January 2024, but Tindall didn’t stop there. Inspired by the simplicity of the results, he and his co-author Dries Sels of the Flatiron Institute and New York University set out to determine why this system could be so easily solved with a classical computer when, on the surface, it appeared to be a very complex problem.
“We started thinking about this question and noticed a number of similarities in the system’s behavior to something people had seen in one dimension called confinement,” Tindall says.
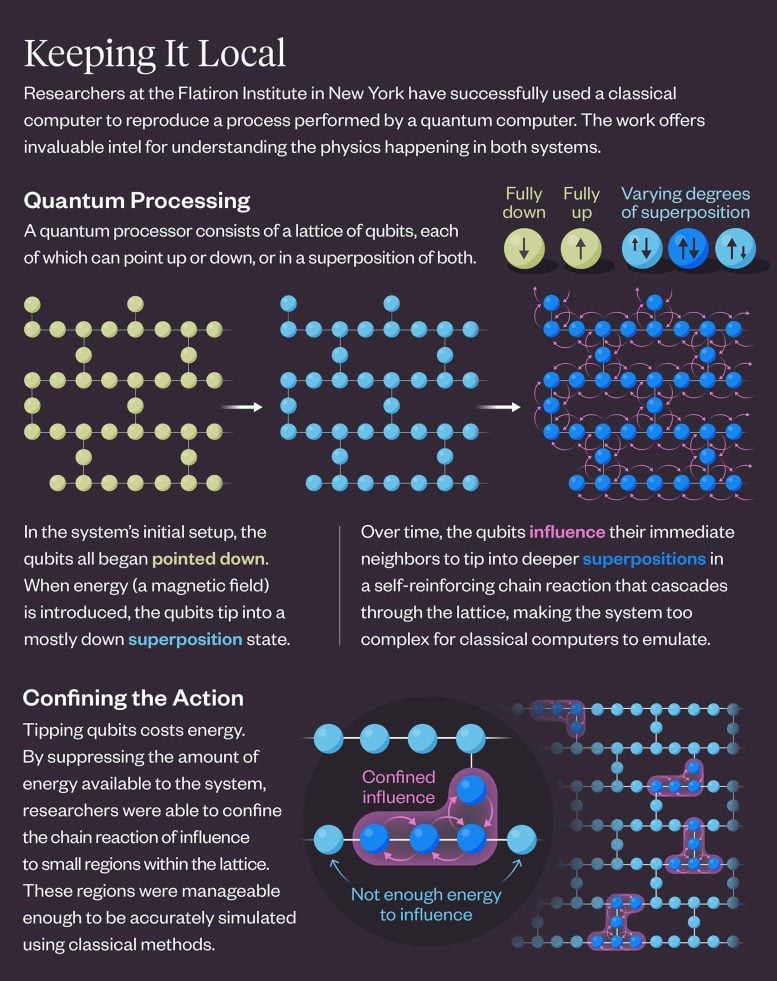
Confinement is a phenomenon that can arise under special circumstances in closed quantum systems and is analogous to the quark confinement known in particle physics. To understand confinement, let’s begin with some quantum basics. On quantum scales, an individual magnet can be oriented up or down, or it can be in a ‘superposition’ — a quantum state in which it points both up and down simultaneously. How up or down the magnet is affects how much energy it has when it’s in a magnetic field.
Limited Entanglement in Closed Systems
In the system’s initial setup, the magnets all pointed in the same direction. The system was then perturbed with a small magnetic field, making some of the magnets want to flip, which also encouraged neighboring magnets to flip. This behavior — where the magnets influence each other’s flipping — can lead to entanglement, a linking of the magnets’ superpositions. Over time, the increased entanglement of the system makes it hard for a classical computer to simulate.
However, in a closed system, there’s only so much energy to go around. In their closed system, Tindall and Sels showed that there was only enough energy to flip small, sparsely separated clusters of orientations, directly limiting the growth of entanglement. This energy-based limitation on entanglement is known as confinement, and it occurred as a completely natural consequence of the system’s two-dimensional geometry.
“In this system, the magnets won’t just suddenly scramble up; they will actually just oscillate around their initial state, even on very long timescales,” Tindall says. “It is quite interesting from a physics perspective because that means the system remains in a state which has a very specific structure to it and isn’t just completely disordered.”
Discovering a Mathematical Model for Confinement
Serendipitously, IBM had, in their initial test, set up a problem where the organization of the magnets in a closed two-dimensional array led to confinement. Tindall and Sels realized that since the confinement of the system reduced the amount of entanglement, it kept the problem simple enough to be described with classical methods. Using simulations and mathematical calculations, Tindall and Sels came up with a simple, accurate mathematical model that describes this behavior.
New Pathways in Quantum Physics
“One of the big open questions in quantum physics is understanding when entanglement grows rapidly and when it doesn’t,” Tindall says. “This experiment gives us a good understanding of an example where we don’t get large-scale entanglement due to the model used and the two-dimensional structure of the quantum processor.”
The results suggest that confinement itself could show up in a range of two-dimensional quantum systems. If it does, the mathematical model developed by Tindall and Sels offers an invaluable tool for understanding the physics happening in those systems. Additionally, the codes used in the paper can provide a benchmarking tool for experimental scientists to use as they develop new computer simulations for other quantum problems.
Reference: “Confinement in the Transverse Field Ising Model on the Heavy Hex Lattice” by Joseph Tindall and Dries Sels, 29 October 2024, Physical Review Letters.
DOI: 10.1103/PhysRevLett.133.180402